 Graph Theory Lessons
Graph Theory Lessons



 Lesson
17: Laces
Lesson
17: Laces

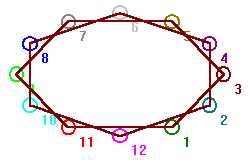
A lace Ln,r,
also known as a circus, is a graph on n vertices x1,x2,...,xn,
with vertex xi adjacent to vertex xj, if j = (i + r) mod n. To see an example in the program
click Graph and Lace and L(n,r). When asked for the number n of vertices
enter 12 and when asked the value r of the lacing, enter 2. This will give
you the graph in figure. 27.
|
|
Fig. 27,
The lace L12,2.
|
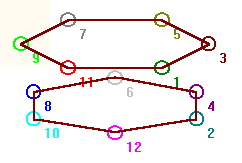
Now click Properties and Components. You will
find that the graph has two components. You can display them by clicking
Picture and Show Components as in figure 28.
|
|
Fig. 28,
The two components of the lace L12,2.
|
-
How many components do the graphs L12,1,
L12,2, L12,3, L12,4,...,L12,11
have?
- How many components do the graphs L25,15 and L20,12
have?
-
In general how many components does Ln,m
have?
-
How many vertices does each component of Ln,m
have?
-
Under what conditions is Ln,m isomorphic
to the circuit C(n)?
We can of course lace the graph more than once. The Lace Ln,r,s
is a graph on n vertices x1,x2,...,xn,
with vertex xi adjacent to vertex xj if j = (i + r) mod n or j = (i + s) mod n. To get Ln,r,s the
procedure is the same as before except that you choose the option L n,r,s .
-
How many components will Ln,r,s
have? [You will have to do a few experiments before you see a pattern].
-
How many vertices will each component of Ln,r,s
have?
 e-mail: C.
Mawata
e-mail: C.
Mawata



 © C. Mawata
© C. Mawata


 Lesson
17: Laces
Lesson
17: Laces

